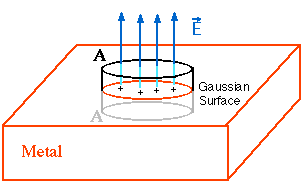
 Consider
a metallic surface having a surface
charge density
Consider
a metallic surface having a surface
charge density  Consider
a metallic surface having a surface
charge density
Consider
a metallic surface having a surface
charge density
placed in a medium of permittivity
The charges are uniformly distributed over the surface of the metal. Here we have only drawn a few inside the Gaussian cylinder, but they are located everywhere on the metal surface.
Now let us apply Gauss' law. Since the lines of force are normal to a conductor, they do not intersect the side surface of the cylinder, thus the flux through these surfaces is zero. The base that is inside the metal also has zero flux, since the field here is zero. So we get
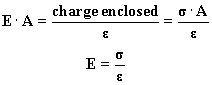
In other words:
The electric field on the surface of the metal is the surface charge density of the metal, divided by the permittivity of the medium.
© MultiMedia Physics 2000