Initial Conditions and Phase

We have earlier encountered the equation of motion for simple harmonic motion as a cosine function. This actually is only true for the special case that the object undergoing simple harmonic motion is at maximum positive displacement at time t = 0. The general expression for SHM is

x(t) = A sin($\omega$t+$\delta$)

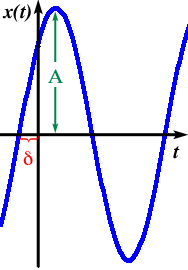
where x(t) is the displacement, $\omega$ is the frequency, and $\delta$ is the phase. The units of phase have to be the same as that of $\omega$t (radians or degrees.) In the figure on the right, you can see what the constants A and $\delta$ mean graphically.

Equations of motion containing sine and cosine expressions are the same except for the phase. In problems dealing with SHM, the phase is determined by the initial conditions.

A simplification that has been used throughout most of this chapter has been to choose the form

x(t) = A cos($\omega$t)

for cases where the displacement is at a maximum at t = 0. However, this is included as a special case of the general equation above, fixing the phase $\delta$ to a value of $\pi$/2. One can understand this fact from the simple trigonometric identity

sin($\omega$t+$\pi$/2) = cos($\omega$t)

You could also select an initial condition where the equation of motion would be

x(t) = A sin($\omega$t)

by choosing a phase of $\delta$ = 0. This could be done by giving the oscillator initially zero displacement but some nonzero value for the velocity.