Example: Sliding Block

Question:

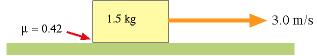
A 1.5 kg block with an initial speed of 3.0 m/s slides to a stop in a straight line on a horizontal surface. If the coefficient of kinetic friction between the block and the surface is 0.42, how much work is done by friction? Compute this result two ways using work-energy considerations and simple kinematics.

Answer:

  • Let's use energy techniques first, note that the final kinetic energy minus the initial is negative, and that there is no change in the potential energy.
W = $\Delta$K = Kf - Ki = 0 - K0 = -mvo2 = - 0.5 $\cdot$(1.5 kg) $\cdot$ (3.0 m/s)2 = - 6.8 J
  • That was easy, now try kinematics and dynamics:
$\sum$Fx = F = m a = $\mu$kmg => a = $\mu$k g \[ \rm \mathbf{v^{2} = v_{0}^{2} + 2ax \Rightarrow x = \frac{v_{0}^{2}}{2a} = \frac{v_{0}^{2}}{2 \mu_{k}g}} \] \[ \rm \mathbf{W = - F $\cdot$ x = - \mu_{k}mg \cdot \frac{v_{0}^{2}}{2 \mu_{k}g}} \] = -mvo2 = - 6.8 J
 (Same result, but much harder to get there.)