Newton's Second Law

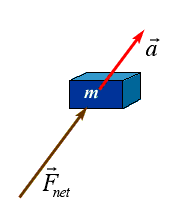
The acceleration of an object is proportional to the net force applied to it. We can write this as:

It is intuitive that if you push twice as hard, you will get twice the acceleration. Please note that the arrows for the acceleration and the force are in exactly the same direction in the drawing on the right, but they do not have the same length. This is a consequence of the above proportionality.

But the mass must also play a role. The relation of mass to acceleration can be stated as

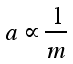
A more massive object is harder to accelerate than a less massive object - also intuitively in line with our everyday experiences!

Combining these two relationships we get

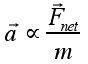
In SI units the proportional sign becomes an equality, which is exactly Newton's second law.

The Second Law

The acceleration of an object is directly proportional to the net force acting on it and inversely proportional to its mass. The direction of the acceleration is in the direction of the applied net force.

(Yes, this is the famous F = ma. Together with E = mc², it is quite possibly the most well-known physics formula.)

Since the above law is a vector relationship, it means that the resulting acceleration vector always points in exactly the same direction as the net-force vector, just as is shown in the figure above.